home

CUTS CD

CUTS is my new CD of compositions, out on the FREEFORM Association label.
cat no FFA-6342, distributed by ReR, Instantjazz, Metamkine, No Man's Land, Soundohm (Italy), Art Into Life (Japan).
This is additional information for listeners who would like to know more about how these pieces were written.

These rambles concern technical aspects of the pieces on this CD. In the first piece, a solo for bass clarinet, I did not apply any mathematical thinking to the time organisation. It is one of a series of pieces in which I set out to write for wind instrument + ring-modulation, and ended up ditching the modulation and finding ways to play some equivalent on the instrument itself. Experimenting with embouchure and breath, I came up with certain harmonics which I then interspersed with occasional multiphonics. I explored some harmonic combinations, wrote down the results, then practiced until I could play them more-or-less accurately, and then went on to record a set of improvised variations, so that the piece keeps returning to the same pitch material and approach, even as it develops. The complete score is downloadable on the SCORES page of this website.



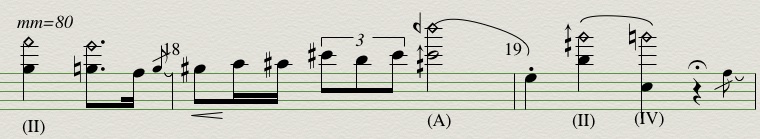
A clarinet is an example of a cylindrical bore instrument closed at one end. The normal resonant modes must have a pressure maximum at the closed end (the mouthpiece) and a pressure minimum near the first open key (or the bell). These conditions result in the presence of only odd harmonics in the sound. This contrasts to the saxophone or oboe, which have a conical bore and hence include the even harmonics. The effect of pressing the register key on a clarinet is therefore to make the frequency jump to the third harmonic, because the second harmonic is absent. Hence the instrument over-blows at the twelfth. Following the system developed by Armand Angster for the Bb clarinet, I number the odd harmonics in Roman numerals. When I write a note with (II), the (II) refers to the fact that the resultant harmonic would be the second of the series of uneven harmonics produced by the clarinet. This would be the fifth harmonic of the fundamental if you counted the even harmonics as well.



However, for the bass clarinet, I was finding the harmonics I wanted using the upper (clarino) register, i.e. with the register key pressed, which causes a slight divergence in the pitching of the harmonics from the ones given by Angster. The (II) is therefore the lowest harmonic to hand for the fingering including the register key. For example, the first note in the extract below, which is the G# just above the treble staff, has the F above that for a (II), and would have the B quarter flat above that for a (III). This G# is, of course, the (I) of C# below the treble staff, which lies in the chalumeau register which is played without the register key. As an example of how I use this, in bar 19 below, which you hear at about 1 minute 12 seconds into the piece, the (II) of the B above the treble clef is immediately followed by the (IV) of the C in the treble clef: what you hear is a faint quarter tone drop at the top, with a drop of a seventh in the bass at the same time.

Now for the second and third pieces. Early in 2011, I got interested in the phenomenology of number. My notes go thusly: On the one hand, maths is a formal ontology, not studying sensible objects but their forms of being. On the other hand, it seems totally concerned with a certain category of actions and these actions involve sensible objects. Numbers, in other words, happen in the first place as a result of acts of counting. Counting involves sub-actions of setting apart, selecting from the broader field, and retaining an awareness of the place or relative order of these sub-acts. Counting produces numerical groups perceived as wholes. It separates out what is counted from what is not counted. (As in: What counts... Count me in, etc.) Counting produces an awareness of an essential generic feature of the whole group. Numerosity is this generic unifying structure of the groups as we see them.



In Hans Freudenthal's Didactical Phenomenology of Mathematical Structure, he jumps very quickly from the question of what number is to the question of what you can do with numbers, and the question is articulated in a way that quickly attaches number to the real world: he talks about length, says nothing about measurement, but explains how two objects having certain lengths have a combined length which is the sum of the lengths. So this is a phenomenology of the mental object that is magnitude, and of the mental activity of using magnitudes. It is a phenomenlogy because it shows you how a mental concept emerges that is otherwise taken for granted. But I am interested in the phenomenology of number itself....the quality of numerosity, the threeness of three... Henri Focillon, in The Life of Forms in Art, wrote that In the life of the mind, there is a region in which forms that are defined with the utmost exactitude nevertheless speak to us in very different languages. I speculate that the action of counting evolves from the counting of actions in a ritual context. Doing something three times would have made that something special, lifted the semantics of the action away from normal functionality. Long before the neolithics started counting their goats, the paleolithics were walking seven times round a tree. Or so my speculation goes. So the presence of number in music does not surprise me.



Phew. In March 2012, everything got worse when my friend Robert Reigle complained to me: why do you use numbers at all in your compositions? I defended myself saying that numbers have a kind of identity beyond their mathematical function, in fact they appear in the human world as distinct characters: think of the three-ness of three. We arrive at a compromise whereby Robert would be happy if I only used numbers up to 5. Five fingers, maybe? Do spiders have a thing about 8? I had already been thinking that in music you could be inaccurate with numbers, using number but in an inexact way, swapping a 6 for a 7 if it seemed propitious at the time. Music is always inaccurate anyway. It measures, but you could always measure more precisely. The way that curves can translate into numbers is a case in point: you could either look at the curve on a grid, and it would give you numbers at the crossing points, and you could change the size of the grid and get fewer or more numbers, or you could locate where the curve changed direction, how fast it went up or down from that point to the next change of direction, and so on. All of which suggests that the relationship between reality and number is contingent: it just depends on how you feel like counting, and where you are going with it. The only restriction seems to be that reality, or our relation to it, is not completely smooth: stuff jumps between quantum states, it can't exist in between them, and its complexities arise from the complicated proportions between whole numbers ( rotations of moons against earths) and not from some implicit fuzziness at base level. The equations that govern regularly vibrating systems only work with whole numbers, and regular vibrations are harmonic, i.e. vibrating at different frequencies occurring in whole number steps.



Later in 2012 I wrote a piece following Robert's rule for Cornelius Dufallo, for solo violin in quarter tones and specified each note in the scale by two of the first five whole numbers. So the fifth quarter tone up from the bass note was 2.1 for example: the first note in the second group of five notes. But what about 5.5? That would be the 25th quarter tone, and there are only 24. Luckily for me, in my pattern this number never occurred. Phew. But the main interest was in the behaviour of groups of numbers that were going through certain processes, with sort of convection currents flowing through them. Causing them to swap their positions around within a pattern. This is really another form of serialism. Strictly speaking 12-tone serialism is highly repetitive because to really have an equal distribution of pitches you need to repeat the series endlessly: I wasn't interested in this, I was interested in patterns that went round until they had done what they had to: it was the character of the process that interested me: was it a kind of folding? Did the pattern turn itself inside out? Did it get to a certain point and then collapse? The joyful moment working on a later composition for clarinet (Ghosts of Departed Quantities) when the pattern suddenly produced a zero, which corresponded to nothing, so that at this point, for the piece to go on, there had to be a crisis of sound, a lurch into another dimension. And this whole approach of using patterns of small numbers had been triggered by the discussion with Robert.



I think my current interest in number answers to a problem that I have working with a more spectral approach to composition: where is the level of abstraction? where is whatever there is about the piece that could have translated into other sounds? What I like about maths, as opposed to say traditional music theory, is the way that you can apply the maths in totally diverse ways to the production of sounds. The two pieces for ensemble on this CD come from a phase in which I wanted to compose the processing of the sounds by different kinds of vibrato, glissando, etc, and leave the choice of pitch undetermined, as well as (mostly) the exact choice of timing. Both these pieces involve the use of the number patterns I have referred to, to decide what instrumental techniques are applied to the sound production, and the order in which different instruments enter, what register an instrument plays in (but not pitch and not exactly when it begins in relation to some measured regular pulse). So as a conductor I am laying down a rather complex pattern of cues, but these durations (with the exception, sometimes, of downbeat accents) are not audible on the surface, but are spaces in which the incidence of sounds is shown graphically in the score in relation to other sounds. (Violin just after trombone, cello just after violin etc) ... The choice of process integrates metaphorically with the kind of meaning the piece has for me whilst I am writing it - which doesn't necessarily belong to the public persona of the piece, but is something I need just to be able to get on with the work of writing it.



The extract from the score on the back of the CD cover corresponds to what is played from 2 minutes 30 seconds into On Earth, the second piece on the CD. In this section piano solo alternates with a trio of harp, violin and trombone, with glass, voice and cello playing sustained chords in the background.There are three systems on the page. The first two systems involve alternation, and the third system belongs to the next section of the piece in which the elements become merged. The numbers that you see written above the systems are durations. The piano has 6 + 4 + 5 + 10, then the trio has 13 + 7, the piano 5, the trio 7 + 3, the piano 7 + 3 + 10 + 5, the trio 6 + 9. Adding up these time-groups and dividing by five gives a set of proportions 5,4,1,2,5,3. Of these, the first belongs to a previous group of 5 numbers, and the remaining numbers make up a group of 5. At the same time other sets of 5 numbers are feeding into the music in the form of a set of 5 different kinds of interference in the sounds ( vibrato, added noise, cracking up, added harmonics, and pitch glissando.) A further set cross-cuts this set by specifying 5 different kinds of sound lay-out ( repeated, staccato, sustain, pointilism, open.) In other parts of the piece, other sets specify the orders of entry of the instruments inside the durations. There are many other complications and variations but all of them involve the same system for sequentially shuffling a row of 5 numbers in such a way as to move the inner numbers out to the edges, and bring the outer numbers in to the centre. This constant motion is the inner motor of the piece.



Ananké, the third piece on the CD is written in a related way, but uses different kinds of shuffling, as it concerns rotational movements that are inherently unstable. Ananké is a goddess of fate, a force in the cosmos, turning and returning relentlessly for ever. Also: a moon of Jupiter, spinning a crazy eccentric orbit, born from collision and probably headed for one. Humans, working off, through, with, imbalances: imbalances productive of change. Humans, polyrhythmic, knowing and unknowing, daughters and sons of Ananké, fretful, forgetful, zapped by cosmic dust - our thoughts washed nightly by moons, surely also catastrophe-born and catastrophe-bound. And the span of a life, finally, perhaps 75 times round the cycle of the earth's tilt, the spin seeming to accelerate as we go on. But there is more: Ananké also represents the principle that the labouring members of society are denied all share in the product of their labour beyond the minimum necessary to keep them labouring. And Ananké is the slave-driver in the Orphic cult. I am referencing George Thomson's Aeschylus and Athens...



So far as composing these pieces goes, between sections you could tighten or loosen the grip of numbers. You would then take that into account in your next moves: each significant change becomes part of a pattern of changes. Rather than giving in to the stability and security offered by the numbers, you see them as giving you a series of problems to be solved in terms of working towards an imagined result. And of course the patterns come to an end, and have to be restarted. Differently.



So far as playing these pieces goes, oddly, or paradoxically, enough, this maths seems to have generated a kind of garden or environment for the musicians to inhabit.